What’s The Square Root Of 4 – The Ultimate Guide!
Mathematics is a language that speaks volumes about the world around us, often unraveling complexities and answering questions that pique our curiosity.
Among its many wonders, the concept of square roots stands out as a fundamental pillar. At its core lies a simplicity that belies its significance.
In this exploration, we delve into the seemingly straightforward yet profoundly insightful question: What is the square root of 4?
Understanding Roots in Mathematics – Here To Know!
Before diving into the specifics of the square root of 4, let’s establish a foundational understanding of what roots are in mathematics. In essence, roots are the inverse operations of exponents.
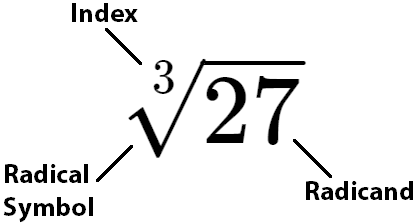
For instance, if we consider a number raised to a power, such as finding the square root involves determining the number that when multiplied by itself, yields the original number,
The Square Root of 4 – Unraveling the Mystery!
Now, turning our attention specifically to the square root of 4, the question seems deceptively simple. Indeed, it is a basic arithmetic operation that even young students encounter early in their mathematical journey. The square root of 4 is, quite plainly, 2. Why is this the case?
To understand why 2 is the square root of 4, we can visualize it geometrically. Imagine a square with sides of four units in length. The area of this square is
4×4=16. Now, to find the length of one side of this square, we seek the square root of 16. Since the sides of a square are identical, the length of one side, which is the square root of the area, is 4. This visual representation provides a tangible understanding of why the square root of 4 is indeed 2.
However, it’s important to note that every positive real number has two square roots: one positive and one negative. In the case of 4, the positive square root is 2, while the negative square root is -2. This stems from the fact that when multiplied by itself, both 2 and -2 yield 4:
Extending the Concept: Complex Roots
While we’ve primarily focused on real numbers thus far, it’s worth mentioning that the concept of square roots extends beyond the realm of real numbers into the domain of complex numbers. Complex numbers consist of a real part and an imaginary part, typically denoted as
When dealing with complex numbers, every non-zero complex number has two square roots. These roots, known as complex square roots, often involve the square roots of both the real and imaginary parts of the number.
Applications of Square Roots – Everything To Know!
The concept of square roots finds application in various fields, ranging from mathematics and physics to engineering and finance. In mathematics, square roots are essential in solving quadratic equations, which frequently arise in algebra and calculus.
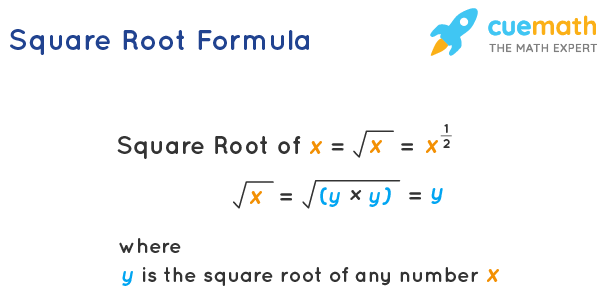
Additionally, they play a crucial role in geometry, particularly in determining the lengths of sides and the areas of shapes.
In physics, square roots appear in numerous formulas, such as those governing motion, electricity, and magnetism. For instance, in classical mechanics, the equation for the speed of an object undergoing uniform circular motion involves the square root of the product of the radius and centripetal acceleration.
Furthermore, square roots are integral to engineering disciplines, where they are utilized in calculations involving forces, stresses, and vibrations.
In electrical engineering, for instance, square roots feature prominently in formulas related to alternating current (AC) circuits and signal processing.
Moreover, square roots have significant implications in finance, particularly in the realm of investment analysis and risk management.
Concepts such as the standard deviation, which measures the dispersion of a set of data points from its mean, involve the calculation of square roots.
Conclusion:
Amidst the myriad marvels of mathematics, the concept of square roots emerges as a cornerstone of fundamental understanding. Beneath its surface simplicity lies a depth of significance that is often overlooked.
FAQ’s:
1. What is the square root of 4?
The square root of 4 is 2. It’s the number that, when multiplied by itself, equals 4.
2. Why is the square root of 4 only 2?
In basic arithmetic, we consider the positive square root by convention. So, for 4, it’s 2. However, -2 is also a valid square root of 4.
3. How do square roots relate to geometry?
Square roots are crucial in geometry for calculating lengths, areas, and volumes of shapes. They help determine side lengths of squares and other geometric figures.
4. What are complex square roots?
Complex square roots extend beyond real numbers into the realm of complex numbers. For instance, for 4, the complex square roots are 2 + 0i and -2 + 0i.
5. Where are square roots used outside of mathematics?
Square roots find applications in various fields such as physics, engineering, and finance. They’re used in formulas for motion, electrical circuits, and risk analysis, among others.
6. Why do we study square roots?
Understanding square roots provides insights into basic arithmetic and lays the groundwork for tackling more complex mathematical concepts. They have practical applications in diverse areas of science and technology.




